This blog notes down all key concepts and properties to remember to be able to solve problems related to number properties. Many GMAT Data Sufficiency & GMAT Problem Solving questions need basic know-how and knowledge of properties so we can infer if sufficient information is available or not. GMAT number properties section needs to be revised at least once.
Even-Odd
There are broadly two kinds of integers, even and odd. 2 is the smallest even number and 1 is an odd number.
- Odd Number: Any integer not divisible by 2 is an odd integer. 0 is not an odd integer. It May or may not be a prime number.
- Even Number: Every even number is divisible by 2. 2 is the only prime integer.
There are some properties between odd and even numbers when operating between them.
- Odd + Odd: The sum of two odd numbers is even.
- Even + Even: The sum of two even numbers is even.
- Odd + Even: This will be an odd number. Odd can be written as even + 1. Even + even + 1 is odd number.
The same logic will apply when even applying subtraction instead of addition. What about operations involving the multiplication of two integers?
- Odd*Odd: The product of two odd numbers is odd.
- Even*Even: The product of two even numbers is even.
- Odd*Even: This will be an even number. The even will have 2 as a factor and therefore, the product will also have 2 as a factor.
What about operations involving the division of two integers? These are only valid if the numbers are divisible.
- Odd/Odd: The division of two odd numbers is odd.
- Even/Even: The division of two even numbers is either even or odd. We need a 2 as a factor for a number to be even.
- Even/Odd: This will be an even number. The resultant will have 2 as a factor and therefore will be even.
Basic Number Properties
- Digits Place: The most basic property is knowing the names of the different places to the left and the right of the decimal. We can move the digits to the left or right by either multiplying by 10 or dividing by 10.
- PEDMAS: While carrying out the operations on an equation. The order of operations is determined in the following way:
- Parenthesis
- Exponents
- Division
- Multiplication
- Addition
- Subtraction
Thereby, the key order is division or multiplication. Whichever comes first on reading from left to right. Similarly for addition and subtraction. Choose the one that comes before when reading left to right.
Fractions
When dealing with fractions, multiplying a constant onto both the numerator and denominator does nothing to the value of the fraction. This means a constant on both numerator and denominator can be cancelled as well.
The addition of fractions will require one to compute the LCM of the denominators. Therefore one can simply add the numerators and compute the ratio.
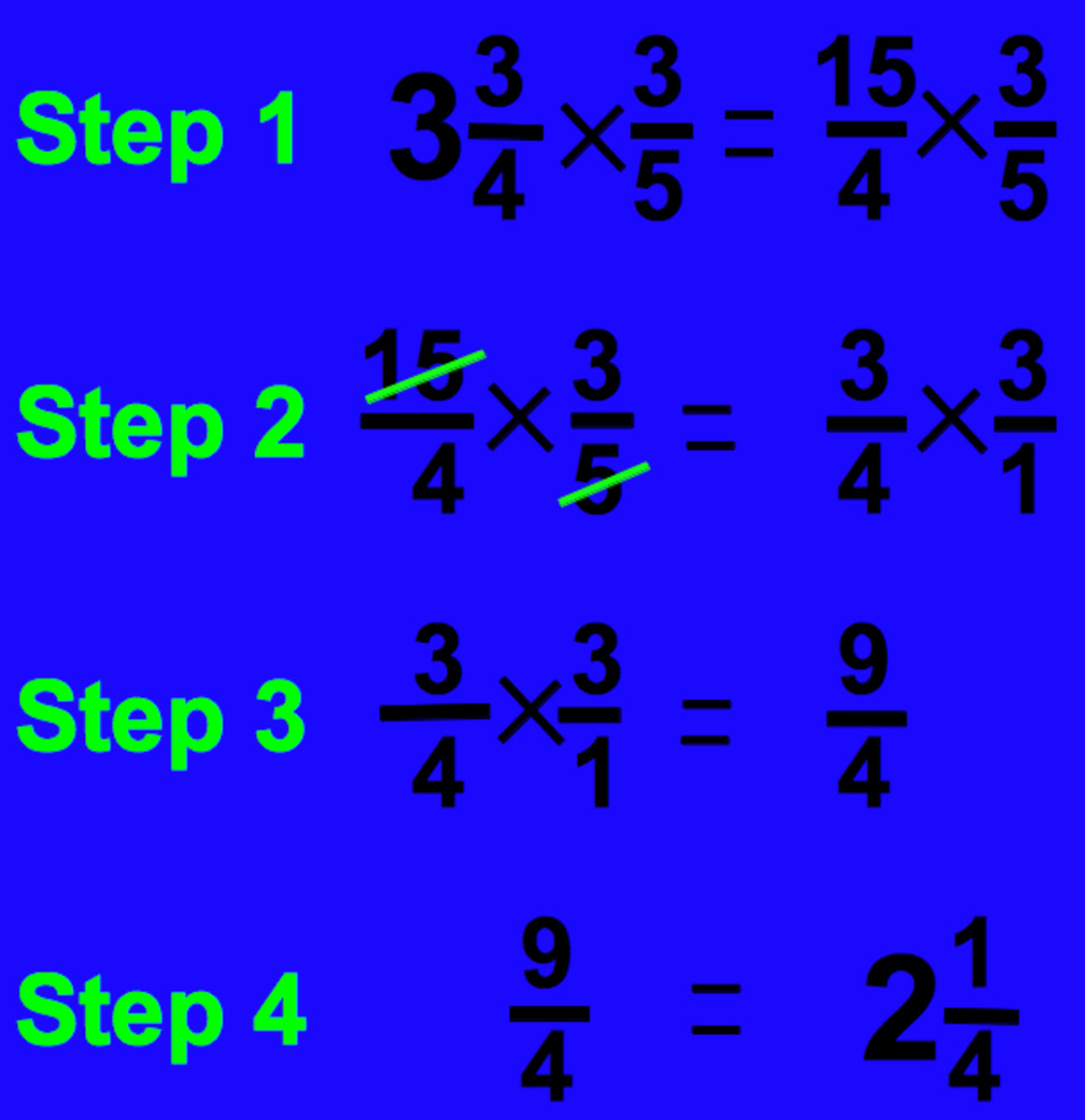
Fractions multiplication is the same as multiplying the numerators and denominators separately and taking the fraction of the product of numerator/denominator. Refer to Step 1 for the conversion of the first fraction to a different form. 3*4+3 = 15
Decimals
The operations for the decimals follows a structure where we anchor the operations around the decimal. So addition, subtraction, multiplication and division, all centre around the decimal. The below infographic does a good job of summarising all parts.
Besides these, there is this concept of terminating and non-terminating fractions. Terminating essentially means, the decimal of the fraction is of a limited length while in the case of non-terminating, the decimal goes along indefinitely.
The trick to determining if a fraction is terminating or not is to check if the denominator is of the form 2^n X 5^m. Where n and m are integers. If the denominator has any factor other than 2 or 5, we will have non terminating fraction.
Estimation (Rounding)
Estimating and approximating values to arrive at the answer fast is key for speed. How much we can approximate is a function of how close or far apart, the answer choices are.
Figure out how far apart the choices are from each other in terms of units, is it the tenths, hundredths or something else. Accordingly round that same unit and compute the answer.
When computing values of fractions. We can approximate by turning both the numerator and denominator to the closest 5 and getting the value. Preferably increase or decrease both the numbers to be closer to the actual value.
To be continued …